1 Introduction
This book contains the full text of all letters from Emil Artin to Helmut Hasse,
as they are preserved in the Handschriftenabteilung of the Göttingen
University Library, written in the years 1923 – 1934. There are 49 such letters.
Unfortunately, the corresponding letters from Hasse to Artin seem to be lost;
Artin was known not to keep many of the letters and papers which he received.
So we have supplemented Artin’s letters by detailed comments where we discuss
their mathematical content, comparing this with a description of the
mathematical environment of Hasse and Artin, of the tendencies of the time, and
of the relevant literature. In this way it will become possible for the reader to
obtain some idea of the content of the corresponding letters from Hasse to Artin
too.
Artin and Hasse were among those who shaped modern algebraic number
theory. They were of the same age, born in the year 1898. They belonged to the
post-war generation of mathematicians who started their university education
towards the end of and immediately after World War I, Artin in Leipzig with
Herglotz (after a brief interlude in Vienna 1917 with Furtwängler) and Hasse in
Marburg with Hensel (after brief interludes in Kiel 1917 with Toeplitz, and
in Göttingen 1918/19 with Hecke). They obtained their Ph.D. in the
same year 1921, and their two dissertations were considered as ground
breaking contributions to number theory. Artin’s thesis contained the
theory of hyperelliptic function fields over a finite field of constants, and he
formulated the analogue of the Riemann hypothesis for those fields which was
later proved by Hasse in the elliptic case and by A.Weil in the case of
function fields of arbitrary genus. Hasse’s thesis contained the Local-Global
Principle for quadratic forms over the rationals which he later, in his
habilitation thesis, generalized to quadratic forms over arbitrary number
fields.
In Germany at that time, one had to pass one’s „Habilitation“ in order to
qualify for the position of professor at university. Both Artin and Hasse
did their Habilitation in short succession, Hasse in 1922 in Marburg and
Artin in 1923 in Hamburg. We have already mentioned above that Hasse’s
Habilitation thesis contains the Local-Global Principle for quadratic forms
over number fields. Artin’s Habilitation thesis is his seminal paper on
his new L-series, which led him, among other results, to his Reciprocity
Law.
The mathematical careers of Artin and Hasse in the 1920s developed quickly
and in remarkably parallel steps, from which one can conclude that they were
considered by the mathematical community as leading scientists of equal, high
standing. Let us explain:
In the fall of 1922 Hasse accepted the position of Privatdozent at the
University of Kiel which had been offered to him by Toeplitz. Actually, Toeplitz
originally wanted to get Artin for this position but the latter was not able to
accept; in a letter dated February 27, 1922 Artin wrote to Toeplitz that he had
already accepted a stipend, for the summer semester of 1922, from Courant in
Göttingen.
At that time Artin was in Göttingen as a post-doc. But a little later, in October
1922, Artin accepted a position in Hamburg offered to him by Blaschke.
And in 1923, after his Habilitation, he became Privatdozent at Hamburg
University.
In 1923 there appeared the first joint paper of Artin and Hasse.
In 1925 Hasse accepted an offer of a position as full professor at
the University in Halle. This time again, Toeplitz had recommended
Artin for this position but, for reasons unknown to us, Artin had
not been taken into consideration by the nomination committee in
Halle.
Shortly afterwards, in early 1926, the University in Münster had to fill a vacancy
of full professor in Mathematics, and the proposal of the Faculty was, first Hasse
and secondly Artin. Since Hasse had just moved to Halle, the position was
then offered to Artin who, however, declined since Hamburg matched
the offer and he was promoted to full professor in Hamburg. Thus now
Artin and Hasse were for a time the youngest Mathematics professors in
Germany.
In 1928, the University of Breslau had to find a successor for the retiring Adolf
Kneser (the father of Hellmut Kneser and grandfather of Martin Kneser). Like
two years earlier in Münster, the Faculty in Breslau also proposed the
names of both Artin and Hasse, but this time in the reverse order: first
Artin and then Hasse. Neither of the two accepted; Artin remained in
Hamburg and Hasse in Halle. In the same year Artin got another offer,
this time from the University of Leipzig. Again he declined. At the same
time A.Fraenkel, who was in Kiel at that time, tried to get Hasse back
from Halle to Kiel. From the Fraenkel–Hasse correspondence one can see
that Fraenkel had tried everything in his power to have the ministry of
education extend an offer to Hasse for a position in Kiel. However the offer
never came since the ministry was of the opinion that, instead, Hasse
should be offered the position in Marburg after the retirement of Kurt
Hensel, Hasse’s academic teacher. Indeed in 1930, Hasse moved from
Halle to Marburg. In the same year, Artin obtained an offer from the
ETH in Zürich as the successor of Hermann Weyl who had moved to
Göttingen .
Again, Artin decided to stay in Hamburg.
We have said above that Artin and Hasse were considered by the
mathematical community as of equal standing, but this does not mean that they
worked closely together, not even that their mathematical interests were identical.
We see from their correspondence that they freely exchanged mathematical ideas
and informed each other about recent results, mostly about class field theory and
Reciprocity Laws which were the prominent topics of their discussion. But at the
same time each of them also followed other lines of interest which are not
mentioned in their letters, and each kept his own distinctive mathematical
style.
Let us point out that both Artin and Hasse belonged to what
Yandell
has called the „Honors Class“, in the sense that each of them had solved one of
the famous problems which Hilbert had presented in the year 1900 in his Paris
lecture.
Artin solved the 17th Hilbert problem which reads:
…ob nicht jede definite Form als Quotient von Summen von
Formenquadraten dargestellt werden kann.
…whether every definite form may be expressed as a quotient of
sums of squares of forms.
Artin obtained the positive answer to this question through the theory
of formally real fields which he had developed jointly with Otto
Schreier.
Hasse had worked on the 11th Hilbert Problem:
…Aufgabe, eine quadratische Gleichung beliebig vieler Variabeln
mit algebraischen Zahlencoeffizienten in solchen ganzen oder
gebrochenen Zahlen zu lösen, die in dem durch die Coefficienten
bestimmten algebraischen Rationalitätsbereiche gelegen sind.
…to solve a given quadratic equation with algebraic numerical
coefficients in any number of variables by integral or fractional
numbers belonging to the algebraic realm of rationality determined
by the coefficients.
Hasse obtained a criterion of solvability by means of his Local-Global-Principle
which permitted the reduction of the question to the local case where it
could be explicitly discussed, thanks to Hensel’s results about the
p-adics.
There is another Hilbert problem whose solution has to be credited jointly to
both Artin and Hasse, namely the 9th problem which concerns class field theory
and reciprocity. The problem reads:
Für einen beliebigen Zahlkörper soll das Reciprocitätsgesetz der
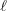 -ten
Potenzreste bewiesen werden, wenn
-ten
Potenzreste bewiesen werden, wenn
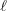 eine ungerade Primzahl bedeutet
und ferner, wenn
eine ungerade Primzahl bedeutet
und ferner, wenn
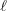 eine Potenz von 2 oder eine Potenz einer ungeraden
Primzahl ist. Die Aufstellung des Gesetzes, wie die wesentlichen
Hülfsmittel zum Beweise desselben werden sich, wie ich glaube,
ergeben, wenn man die von mir entwickelte Theorie des Körpers der
eine Potenz von 2 oder eine Potenz einer ungeraden
Primzahl ist. Die Aufstellung des Gesetzes, wie die wesentlichen
Hülfsmittel zum Beweise desselben werden sich, wie ich glaube,
ergeben, wenn man die von mir entwickelte Theorie des Körpers der
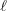 ten Einheitswurzeln
und meine Theorie
des relativ-quadratischen Körpers in gehöriger Weise verallgemeinert.
ten Einheitswurzeln
und meine Theorie
des relativ-quadratischen Körpers in gehöriger Weise verallgemeinert.
For any number field the law of reciprocity is to be proved for the
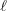 -th power residues, when
-th power residues, when
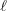 denotes an odd prime, and further
when
denotes an odd prime, and further
when
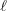 is a power of 2 or a power of an odd prime. The law, as
well as the means essential to its proof, will, I believe, result from
suitably generalizing the theory of the field of
is a power of 2 or a power of an odd prime. The law, as
well as the means essential to its proof, will, I believe, result from
suitably generalizing the theory of the field of
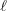 -th roots of unity
which I developed, and my theory of relatively quadratic fields.
-th roots of unity
which I developed, and my theory of relatively quadratic fields.
The first part, for an odd prime
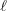 , had been solved by
Furtwängler and
also by Takagi.
But for the case of higher prime powers there was no general approach in sight
before Artin’s Reciprocity Law had opened the way. The implementation of
Artin’s result for the deduction of explicit formulas for the Reciprocity Laws, in
case of power residues for an arbitrary exponent, is due to Hasse; it is published
finally in the second part of his Klassenkörperbericht. The Artin–Hasse
correspondence documents that and how they cooperated to achieve this aim.
The „suitable generalization“ of Hilbert’s theory of relatively quadratic fields has
turned out to be precisely Takagi’s class field theory, crowned with Artin’s
Reciprocity Law.
, had been solved by
Furtwängler and
also by Takagi.
But for the case of higher prime powers there was no general approach in sight
before Artin’s Reciprocity Law had opened the way. The implementation of
Artin’s result for the deduction of explicit formulas for the Reciprocity Laws, in
case of power residues for an arbitrary exponent, is due to Hasse; it is published
finally in the second part of his Klassenkörperbericht. The Artin–Hasse
correspondence documents that and how they cooperated to achieve this aim.
The „suitable generalization“ of Hilbert’s theory of relatively quadratic fields has
turned out to be precisely Takagi’s class field theory, crowned with Artin’s
Reciprocity Law.
We do not know when Artin and Hasse met for the first time. It may have
been at the annual meeting of the German Mathematical Society (DMV)
in September 1922 in Leipzig which both attended. Artin presented a
talk on a problem from analysis and geometry which had arisen in a
correspondence with his academic teacher Herglotz in Leipzig. Hasse did
not give a talk, he just accompanied his academic teacher Hensel to the
meeting.
Artin and Hasse certainly met several times during the winter semester
1922/23. As said above, at that time Artin was in Hamburg and Hasse was in
Kiel. The towns of Hamburg and Kiel in northern Germany are not too far apart
from each other, about 100 km. The mathematicians in Kiel often went to the
colloquium in Hamburg which was led by Blaschke and Hecke. On those occasions
Hasse and Artin met and there developed a close mutual exchange of
mathematical ideas. Artin learned from Hasse how the p-adic methods of Hensel
could be successfully applied to number theoretical problems, and Hasse was
informed by Artin about the two great papers by Takagi on class field theory and
on Reciprocity Laws.
For both Artin and Hasse the encounter with Takagi’s papers turned out to be
an important stimulus for their future work. They immediately realized the
enormous potential of the main discovery of Takagi, namely that every abelian
extension of a number field is a class field. In fact their whole correspondence,
which centers around class field theory and reciprocity, can be regarded as
reflecting their critical preoccupation with Takagi’s papers, and their attempt to
further simplify, streamline and complete class field theory and to put it to work
in number theory.
In the Takagi biography by Honda [Hon76] the story is told how Artin and
Hasse got hold of a copy of Takagi’s papers. Honda reports that Takagi had heard
about a brilliant young mathematician in Göttingen with the name of Siegel.
Subsequently Takagi sent to Siegel a reprint of his first great paper [Tak20] on
class field theory. And Honda continues:
One day, when Siegel was talking with Artin about number fields,
he took out the reprint which Takagi had sent to him, and
persuaded Artin to read it. This was at the beginning of 1922.
Artin borrowed the reprint from Siegel. He spent three weeks in
reading it through. Later, in 1962, he told the present author
[Honda]: I felt strong admiration for it. It was not difficult to
understand, since it was written very clearly.
Somewhat later in Hamburg, Artin showed Takagi’s papers to Hasse. This incident
was recalled when Honda interviewed Hasse:
In 1923 Artin urged him [Hasse] to read the two papers of
Takagi. Reading the first paper, Hasse was deeply fascinated by its
generality, its clearness, its effective methods, and its wonderful
results. He was given an even stronger inspiration by the second
paper.
Takagi’s second paper [Tak22] deals with the Reciprocity Law for power residues of
prime exponent.
Thus, by 1923 there were three young mathematicians in Germany who had
read and appreciated Takagi’s papers on class field theory: Siegel, Artin and
Hasse. Each of them immediately started to integrate Takagi’s results into his
work with striking results, and in this way these results became quickly known
among mathematicians – although 3 years prior to this Takagi had not met with
any visible response when he presented his paper at the International
Mathematical Congress in Straßburg where German mathematicians had not
been admitted.
As to Artin, already his 1923 paper on Galois L-series relied
heavily on Takagi. Although his main idea, namely the construction of
L-series for Galois extensions, was evidently inspired by the work of
Hecke , there
was one important point where Artin had to use Takagi. This was when Artin tried to
identify his new L-series in the abelian case with the classical L-series of Dirichlet and
Weber .
In order to do this, he had to use Takagi’s class field theory which implied that
for any abelian extension of number fields there exists an isomorphism of the
corresponding ray class group of the base field with the Galois group. But this
was not quite sufficient for Artin, for he needed the fact that there is a canonical
isomorphism given by associating to every unramified prime of the base field its
Frobenius automorphism in the Galois group. Artin could not yet prove this in
1923. Only in the special case of a cyclic extension of prime degree (and also of an
extension composed of these) could he extract this from Takagi’s papers. But
that was only temporary. Four years later in 1927, Artin could prove
his general Reciprocity Theorem for arbitrary abelian extensions, thus
putting his new L-series on a solid base and at the same time completing
Takagi’s class field theory. This important result was hailed by Takagi
as
one of the most beautiful results of algebraic number theory.
In fact, Artin’s Reciprocity Theorem completely changed our understanding of class
field theory which today is seen as the key to much of algebraic number theory. In
the Artin–Hasse letters we can observe the exciting story of its discovery, and how
it was put to work immediately.
As to Hasse, he realized that Takagi’s papers could be put to use in
the study of explicit Reciprocity Laws which was his main interest in
those days. Already on April 23, 1923 he reported to his academic teacher
Hensel:
…Außerdem habe ich gerade die Ausarbeitung eines Kollegs über die
Klassenkörpertheorie von Takagi vor, die ich mit unseren Methoden
sehr schön einfach darstellen kann.
…Furthermore, I am just preparing the manuscript for a course
on Takagi’s class field theory, which I can develop quite nicely
with our methods.
Thus Hasse did what mathematicians often do, namely he gave a lecture course since
he wished to learn more about the topic. When he mentions „our methods“ then
he means the p-adic methods of Hensel which he, Hasse, was endeavoring to put
into their proper place in algebraic number theory. At the time Hasse
was mainly concerned with the theory of norm residues which Hensel
had started to investigate with p-adic methods. Norm residues play an
important role in class field theory, and so it seems to us that Hasse, in this
letter to Hensel, meant that he can develop the theory of norm residues
„quite nicely with our methods“. This is evident from Hasse’s papers
from those years, among them the joint paper of Artin and Hasse which
is discussed in the first 5 letters of their correspondence. (Later in the
early 1930s, Hasse was indeed able to develop class field theory with
essential use of p-adic methods but we have no indication whether already at
the time of this letter, in 1923, he had definite ideas on how to achieve
this.)
Hasse delivered his lecture course on Takagi’s class field theory in Kiel
in the
summer semester 1924. The notes for this course were composed by Reinhold
Baer.
They provided the basis for Hasse’s famous report on Takagi’s class
field theory which he had been asked to present at the DMV meeting
in Danzig in September 1925, and whose Part I went into print in
1926 .
This article became known as „Klassenkörperbericht“ and was regarded in line
with Hilbert’s „Zahlbericht“ of 1897. Hasse’s report was not meant to replace the
Zahlbericht, as is sometimes claimed. Hasse’s aim was to amplify the latter by a
survey of Takagi’s class field theory; he wished to give a useful guide
for those who wanted to study the details, so as to avoid unnecessary
detours.
Let us cite a postcard from Bessel-Hagen to Hilbert, dated August 17, 1926,
as an example of the reception of the Klassenkörperbericht:
…In dem vor wenigen Tagen erschienenen Hefte des Jahresberichtes
der D.M.V. befindet sich ein Bericht von Hasse über die
Klassenkörpertheorie, der so vorzüglich klar geschrieben ist und
den ganzen Aufbau der Theorie mit einem nur die Hauptgedanken
enthaltenden Skelett der Beweise so wundervoll herausschält, daß
die Lektüre ein wahres Vergnügen ist und für das Eindringen in die
Theorie jetzt wirklich alle Schwierigkeiten aus dem Wege geräumt
sind …
…A few days ago there appeared the latest issue of the
Jahresbericht D.M.V. which contains a report by Hasse on class
field theory, and which is written in excellent clarity. The design
of the whole theory is wonderfully uncovered by presenting the
main ideas only while the proofs are reduced to their skeletons.
Reading this article is a real pleasure; now all obstacles are
eliminated which may have hampered access to the theory…
The impact of Hasse’s report was remarkable. Since the proofs in the report
were „reduced to their skeletons“ (as Bessel-Hagen wrote), Hasse added an
additional Part Ia which, responding to demand, contained full proofs. Now a
whole generation of mathematicians started to learn class field theory through
Hasse’s Klassenkörperbericht. Their names include Claude Chevalley, Jacques
Herbrand, Max Deuring, Arnold Scholz, Olga Taussky, Shokichi Iyanaga, Max
Zorn, perhaps Hermann Weyl, and many more, not to forget Emmy Noether.
Whereas formerly class field theory was the topic of a select few, Hasse’s report
brought about its „popularization“ among mathematicians. This had the effect
that during the next years the proofs of class field theory quickly became
streamlined, simplified and shortened. Artin and Hasse took active part in
this development; their correspondence provides ample witness for this.
Within a decade, and finally with Chevalley’s idea to use ideles instead
of ideals, class field theory got a new look which was considered more
natural.
Subsequent to Parts I and Ia of the Klassenkörperbericht, there followed
Part II which contained the derivation of explicit Reciprocity Laws on the
basis of Takagi’s class field theory. Hasse had almost completed Part II
when he obtained the information about the successful proof of Artin’s
Reciprocity Law. This caused Hasse to completely rewrite Part II where
now he included Artin’s result and used it as a basis to derive all known
Reciprocity Laws for power residues – in the spirit of Hilbert’s 9th problem for
arbitrary exponents. The story of this is mirrored in the Artin – Hasse
correspondence.
The progress of work in
Mathematics
depends, as we all know, on the facilities for communication between
mathematicians. Publication of papers is one way of communication but this is
usually the final step only; most mathematicians prefer some amount of
communication along the way, during work in progress.
Hasse’s main medium of communication was letters. Hasse was an
ardent letter writer. The Artin file is only one of many others in the Hasse
legacy at the Handschriftenabteilung in Göttingen. Besides numerous
manuscripts and notes there are more than 1600 letter files in the Hasse
legacy. Of course, not all of them are of the same level of interest for the
mathematician as is the Artin file, or the Noether file which we edited some time
ago. But many are quite interesting, and in the future we intend to edit
more from the Hasse legacy. Unfortunately Hasse’s own letters, when
handwritten, are mostly lost whereas the majority of preserved letters
are from his correspondence partners and addressed to him. But as in
the case of Artin’s or Noether’s letters, by carefully reading the replies
and considering the mathematical environment of Hasse at the time, one
may be able to get a fair picture of how he worked, of his main ideas,
aims and hopes, of his relation to colleagues and friends, and about his
personality. His letters contain not only information about his results; he freely
and openly talked about his ideas and the attempts to realize them, and
conversely he asked for the opinions and advice of his correspondence partners.
There was no hiding information in the back, and he never brought up
questions of priority. We can observe all this in his correspondence with Artin
too.
Artin, on the other hand, was not as fond of letter writing; his main medium
of communication was teaching and conversation: in groups, seminars and in
smaller circles. We have many statements of people near to him describing
his unpretentious way of communicating with everybody, demanding
quick grasp of the essentials but never tired of explaining the necessary.
He was open to all kind of suggestions, and distributed joyfully what
he knew. He liked to teach, also to young students, and his excellent
lectures, always well prepared but without written notes, were hailed for
their clarity and beauty. When he wrote letters then most of the time it
was in response to a letter received, and not always quickly. This we
also observe here. Several times he apologizes to Hasse for his delay in
answering, pretending other activities as the cause, but on the whole
giving the impression that it took him some effort to take up the pen and
write.
In this situation it is quite interesting to read the letters between
these two mathematicians which were quite different in temperament,
different in mathematical style and different in their attitude towards
letter writing. The fact that such an extensive correspondence had come
about, sometimes with very high exchange frequency, is due to the fact
that they had something to say to each other, upon a subject by which
they were both fascinated. We may add that both shared similar ideas of
mathematical beauty which they strove to realize in their work as much as
possible.
In our time there are numerous workshops, meetings, conferences, symposia,
colloquia etc. where people can exchange their knowledge and opinions, the year
round all over the globe. And those who cannot attend may use e-mail. It is lucky
that Artin and Hasse lived at a time when letter writing was still widely in
use, and also that at least one of them, Hasse, had been able to save his
correspondence files. This provides us with first hand knowledge about
mathematical developments which could have been gathered only partially from
the published papers.
The exchange of letters between Artin and Hasse did not proceed evenly;
sometimes the letters followed each other in short succession while sometimes
there were years with no letters.
The first 5 letters were written within a week, more precisely between July 7
and 12, 1923. Some time earlier Hasse had visited Hamburg and given a
colloquium talk on his new results about the explicit Reciprocity Laws; this was
on March 1, 1923. On that occasion Artin and Hasse got into a discussion
about the so-called 2nd supplement to the Law of Reciprocity, in case of a
prime exponent
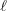 , and they had agreed to continue this discussion when
Artin would visit Hasse in Kiel. Artin’s letters were meant to prepare
this visit which took place on the weekend July 14-16, 1923. As a result
there emerged their first joint paper, which appeared 1925 in Crelle’s
Journal.
, and they had agreed to continue this discussion when
Artin would visit Hasse in Kiel. Artin’s letters were meant to prepare
this visit which took place on the weekend July 14-16, 1923. As a result
there emerged their first joint paper, which appeared 1925 in Crelle’s
Journal.
There followed a period of 2 years without any letter exchanged. This does
not mean that there was no communication between Artin and Hasse. On the
contrary: Hasse visited frequently the Hamburg seminar and so there were many
occasions for discussion and exchange of ideas, on reciprocity and other topics. In
Hasse’s mathematical diary he refers several times to discussions with
Artin.
This period came to an end when Hasse got a professorship in Halle, which
was in the summer semester 1925. In the fall of that year Artin wrote again,
telling that he had returned to number theory from what he called „going astray
on topological roads“ (auf topologischen Abwegen). Apparently Artin wished
to take up again his discussion with Hasse on reciprocity, looking for
ideas towards a proof of his general Reciprocity Law which, as we have
mentioned above already, he had formulated already in 1923 in his L-series
paper.
The breakthrough came in 1927. Artin proudly informed Hasse on July 17,
that recently in a lecture course he had given in Hamburg, his efforts had been
successful and he had obtained a proof. Hasse was excited and replied
immediately, and next day already Artin sent him the full proof. There followed a
period of intense mutual correspondence. Within three weeks Artin wrote 7
letters to Hasse and we may safely assume that there were at least as many
letters from Hasse to Artin. They discussed the implications of Artin’s
Reciprocity Law, in particular the principal ideal theorem, the class field tower
problem and the possible generalization of class field theory from the abelian case
to arbitrary Galois extensions. Hasse was particularly interested in how to
use Artin’s Reciprocity Law for the derivation of explicit Reciprocity
Laws for power residues. Now, thanks to Artin’s result, they could be
obtained for an arbitrary exponent, just as Hilbert had envisaged in his Paris
lecture.
Their correspondence continued at a little slower pace, but still on a high
mathematical level, until November 1932. For the reader of these letters there
unfolds the exciting story of the emergence of new insights into class field theory,
including the Local-Global-Principle for simple algebras and its use for a new
proof of Artin’s Reciprocity Law. We see how Hasse, following a question of
Artin, discovered local class field theory, first as a consequence of Artin’s global
Reciprocity Law but later, on the suggestion of Emmy Noether, on a purely
local basis. Their attempts to generalize class field theory to the case of
non-abelian Galois extensions failed but this led to the beginning of algebraic
cohomology which later culminated in the Artin-Tate version of class field
theory.
In addition, we see the emergence of Part II of Hasse’s Klassenkörperbericht
developing all known reciprocity formulas and much more, based on Artin’s
Reciprocity Law. This book constitutes a monumental work marking the
completion of an era having its roots deep in the past, back to the early 19th
century. When Artin got the proof sheets he replied to Hasse that he had read it
„with great pleasure“ (mit großem Vergnügen) – and he was promptly inspired to
look for explicit formulas for the local contributions of his L-series at the ramified
primes; this he had avoided in his first L-series paper and it did not appear in
Hasse’s book. From this emerged Artin’s famous paper on conductors and
the structure of the discriminant of Galois extensions – in close contact
with Hasse whose contribution led to what was later called the Hasse-Arf
theorem.
Hecke wrote later about the Klassenkörperbericht, in a letter to Hasse dated
November 16, 1938:
Ich
habe kürzlich wieder einmal eingehend Ihren Klassenkörper-Bericht
studieren müssen und bin wieder ganz von Bewunderung erfüllt, wie
Sie diesen riesigen Stoff gemeistert und gestaltet haben.
Lately I have had occasion to look again into details of your class
field report, and again I am filled with admiration how you have
mastered and structured this enormous amount of material.
In November 1932, Hasse gave a colloquium talk in Kiel on the number of solutions
of binary diophantine congruences, in generalization of results of Davenport and
Mordell. Immediately after this he followed an invitation by Artin to Hamburg and
gave a talk at Artin’s seminar on the same topic. At this occasion Artin reminded
him
that the problem was essentially equivalent to the Riemann hypothesis for
hyperelliptic function fields over finite fields which Artin had stated in his
Ph.D. thesis. Thereafter it took Hasse less than three months to arrive at the
proof in the case of elliptic fields. This happened at the end of February
1933.
We are inclined to believe that Hasse had informed Artin about this result since,
after all, it had been Artin who had put Hasse on the track with the zeta function
for function fields. However, we have found no letter from Artin to Hasse in the
year 1933.
But one year later, on January 17, 1934 Artin invited Hasse again to
Hamburg and wrote:
Sie könnten sprechen worüber Sie Lust haben. Vielleicht die schönen
Ergebnisse über die Riemannsche Vermutung? Sie sind doch das
schönste, was seit Jahrzehnten gemacht worden ist. Meine Hörer
würde das sehr interessieren.
You can talk about anything you like. Perhaps the beautiful results
on the Riemann hypothesis? These belong to the most beautiful
things which have been done in decades. The people in my seminar
would be very interested in this.
Of course, Artin did not mean the classical Riemann hypothesis but its analogue for
function fields over finite base fields. In a follow-up letter a few days later Artin
wrote:
Ich bin ganz begeistert von Ihren neuen Ergebnissen und ungeheuer
gespannt.
I am thrilled about your new results and I am immensely curious
about them.
Hasse accepted Artin’s invitation and gave a compact lecture course of one week in
Hamburg, consisting of 4 two-hour lectures, between February 5 and 9, 1934. He
reported about his new method of constructing the endomorphism ring of an
elliptic function field in characteristic p, and how to prove the Riemann
hypothesis by studying the Frobenius operator. Thereafter Hasse wrote to
Davenport:
Hamburg was a full success from every point of view …From
what Artin and I found when considering the possibilities of
generalisation to higher genus, it becomes a matter of patience to
do this.
But it turned out that it took more than patience to arrive at a proof of the Riemann
hypothesis for function fields of arbitrary genus.
We see that Artin and Hasse at that time still vividly discussed mathematical
problems of common interest. However, there were no more letters exchanged. We
do not know the reason for the ensuing silence between the two. One
explanation which offers itself is the deterioration of the political situation in
Germany which had consequences also in academic life. Artin seemed to be
worried because his wife Natascha was of Jewish origin (her father was
Jewish) and she had to suffer the harassments of the time. Hasse was deeply
concerned about the enforced exodus of so many mathematicians. He
considered this as a fatal loss for Germany and did what he could do to act
against this, but without much success. When in 1937 Hasse heard about
plans of Artin to emigrate, he tried, on the one hand to obtain better
and safe working conditions for Artin from the ministry of education,
and on the other hand to persuade Artin to stay in Germany. He could
not succeed under the circumstances and the Artins left Germany in
1937.
As said above, we have supplemented Artin’s letters by detailed comments.
We have given these comments in the language of Artin and Hasse, i.e.,
in German. Short comments are added in the form of footnotes to the
respective letter. More detailed comments are to be found immediately
after the letter with specific headings, which also show up in the table of
contents.
Most of the letters deal with class field theory and with Reciprocity Laws for
power residues. In order to understand the letters it is necessary to recall the
state of the art at the time, and the terminology which was used by Artin and
Hasse. Therefore we have included in the Vorspann two explanatory sections, one
for the Reciprocity Laws of power residues (section 4) and the other for class field
theory (section 5).
Also, we have included in the Vorspann two documents which contain
personal recollections to Artin and to Hasse respectively. For Artin, we have
chosen the obituary written by Hans Zassenhaus, Artin’s doctoral student in
Hamburg (section 2). For Hasse, there is in section 3 a report about the early
work on the present edition, and this contains personal recollections to Hasse. We
believe that those two sections may help the reader to obtain a lively picture of
the personalities of Artin and of Hasse whose mathematical ideas he will find in
their letters.
The Zeittafel in section 6 may be useful to the reader, as an overview of the
development of ideas and results which are discussed in the letters.
ACKNOWLEDGEMENT: As reported in section 3, the present book is the
outcome of many years’ work (with interruptions), based on the first
preliminary version back in 1981 [Fre81a]. We would like to thank all
people who have given us their advice and encouragement during the
writing of the book, and who have helped us with critical comments. Above
all we would like to mention Franz Lemmermeyer who has followed the
preliminary versions of the book and has lent us his invaluable advice and
detailed historical knowledge in order to straighten up many important
points. Patrick Morton has read the introduction and been of help to
streamline our English. Last but not least we are glad that the Deutsche
Forschungsgemeinschaft and the Möllgaard-Stiftung have provided financial
support.
We hope that the reader will enjoy the book as we have enjoyed our work.
Nevertheless, as in any work of this kind and size, there may have occurred errors,
misprints, omissions or other shortcomings. Any comment or correction will be
welcomed.
Günther Frei
Peter Roquette
![]() -ten
Potenzreste bewiesen werden, wenn
-ten
Potenzreste bewiesen werden, wenn
![]() eine ungerade Primzahl bedeutet
und ferner, wenn
eine ungerade Primzahl bedeutet
und ferner, wenn
![]() eine Potenz von 2 oder eine Potenz einer ungeraden
Primzahl ist. Die Aufstellung des Gesetzes, wie die wesentlichen
Hülfsmittel zum Beweise desselben werden sich, wie ich glaube,
ergeben, wenn man die von mir entwickelte Theorie des Körpers der
eine Potenz von 2 oder eine Potenz einer ungeraden
Primzahl ist. Die Aufstellung des Gesetzes, wie die wesentlichen
Hülfsmittel zum Beweise desselben werden sich, wie ich glaube,
ergeben, wenn man die von mir entwickelte Theorie des Körpers der
![]() ten Einheitswurzeln7
und meine Theorie8
des relativ-quadratischen Körpers in gehöriger Weise verallgemeinert.
ten Einheitswurzeln7
und meine Theorie8
des relativ-quadratischen Körpers in gehöriger Weise verallgemeinert.
![]() -th power residues, when
-th power residues, when
![]() denotes an odd prime, and further
when
denotes an odd prime, and further
when
![]() is a power of 2 or a power of an odd prime. The law, as
well as the means essential to its proof, will, I believe, result from
suitably generalizing the theory of the field of
is a power of 2 or a power of an odd prime. The law, as
well as the means essential to its proof, will, I believe, result from
suitably generalizing the theory of the field of
![]() -th roots of unity
which I developed, and my theory of relatively quadratic fields.
-th roots of unity
which I developed, and my theory of relatively quadratic fields.